Ideal clearance. Ideal bearing life.

Calculating Bearing Clearance

Internal clearance is one of the most important factors affecting bearing performance within pump applications. The bearing “internal clearance” is the relative movement of the outer and inner rings when they are lightly pushing in opposite directions. Movement in the diametrical direction is defined as radial clearance, while movement in the shaft’s direction is axial clearance.

Internal clearance is critical to bearing performance for multiple reasons. The amount of clearance influences the load distribution in a bearing, which ultimately affects its life. It also influences bearing running noise and vibration. In addition, it can influence whether the rolling elements move by rolling or sliding motion.

Normally, bearings are installed with interference for either the inner or outer ring, and this leads to its expansion or contraction which causes a change in the clearance. During operation, the bearing will increase until it reaches saturation temperature. However, the temperature of the inner ring, outer ring, and rolling elements are all different from each other, and this temperature difference changes the clearance (Figure 1). In addition, when a bearing operates under load, an elastic deformation of the inner ring, outer ring, and rolling elements also leads to a change in clearance. Quantifying all of these changes can make calculating bearing internal clearance a very complex task.

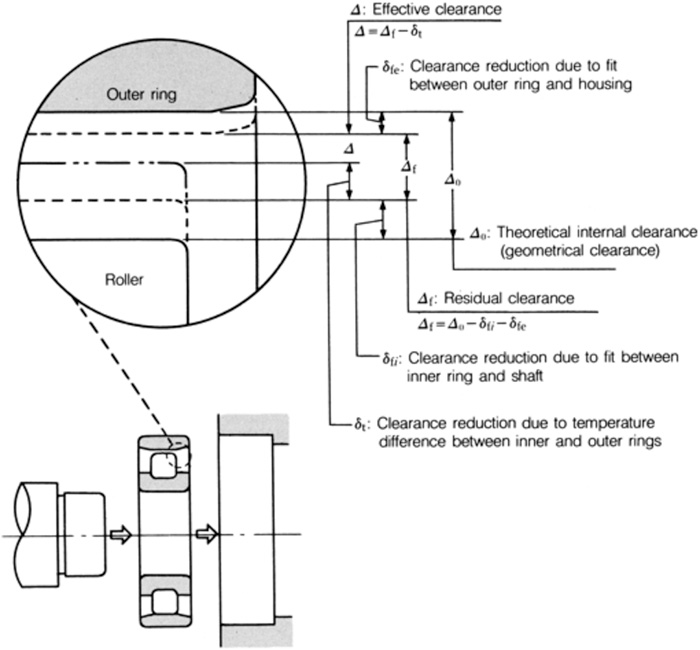Figure 1. Changes of radial internal clearance of roller bearing

So what is the ideal clearance? Before considering this question, first the following different types of clearance will be defined:

Measured Internal Clearance (Δ₁)
This is the internal clearance measured under a specified measuring load, and can be called “apparent clearance.” This clearance includes the elastic deformation (δ_FO) caused by the measuring load.

Δ₁ = Δ₀ + δ_FO

Theoretical Internal Clearance (Δ₀)
This is the radial internal clearance which is the measured clearance minus the elastic deformation caused by the measuring load.

Δ₀ = Δ₁ + δ_FO

δ_FO is significant for ball bearings, but not for roller bearings where it is assumed to be equal to zero, and therefore Δ₀ = Δ₁

Residual Internal Clearance (Δ_f)
This is the clearance left in a bearing after mounting it on a shaft and in a housing. The elastic deformation caused by the mass of the shaft, etc. is neglected. Assuming the clearance decrease caused by the ring expansion or contraction is δ_f, then:

Δ_f = Δ₀ + δ_f

Effective Internal Clearance (Δ)
This is the bearing clearance that exists in a machine at its operating temperature, excluding the elastic deformation caused by load. In other words, this is the clearance when considering only the changes due to bearing fitting δ_f and temperature difference between the inner and outer rings δ_t. The basic load ratings of bearings apply only when the effective clearance Δ=0.

Δ = Δ_f − δ_t = Δ₀ – (δ_f + δ_t)

Operating Clearance (Δ_F)
This is the actual clearance when a bearing is installed and running under load. Here, the effect of elastic deformation δ_F is included as well as fitting and temperature. Generally, the operating clearance is not used in the calculation.

Δ_F = Δ + δ_F

The most important bearing clearance is the effective clearance. Theoretically, a bearing with a slightly negative effective clearance Δ will have the longest life. A slightly negative clearance (or preload) will actually become positive under the influence of bearing load. However, it is impossible to make the clearance of all bearings to the ideal effective clearance, and one must consider the geometrical clearance Δ₀ in order to achieve a zero or slightly negative effective clearance minimum value. To calculate this value, one needs to know both the clearance reduction caused by the interference of the inner ring and outer ring D_f and clearance change caused by the temperature difference between inner ring and outer ring D_t.

Calculating residual internal clearance after mounting

When the inner ring of a bearing is press fit onto a shaft, or when the outer ring is press fit into a housing, the radial internal clearance will naturally decrease due to the resulting expansion or contraction of the bearing raceways. Generally, most pumps have a rotating shaft which requires a tight fit between the inner ring and shaft and a loose fit between the outer ring and housing. In these cases, only the effect of the interference on the inner ring needs to be considered.

An example calculation is shown below for a 6310 single row deep groove ball bearing. The shaft tolerance used is K5, while the housing is H7. The interference fit is applied only to the inner ring.

Shaft diameter, bore size and radial clearance are the standard bearing measurements. Assuming that 99.7% of the parts are within tolerance, the mean value (m_Δf) and standard deviation (σ_Δf) of the internal clearance after mounting (residual clearance) can be calculated. Measurements are given in units of millimeters (mm).

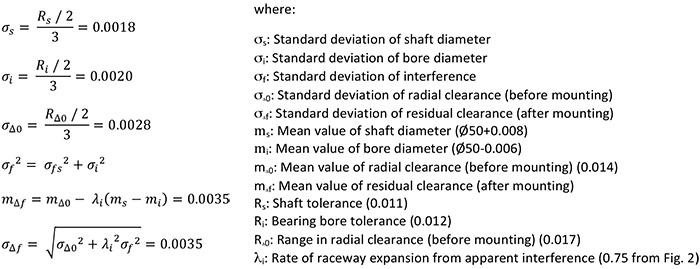
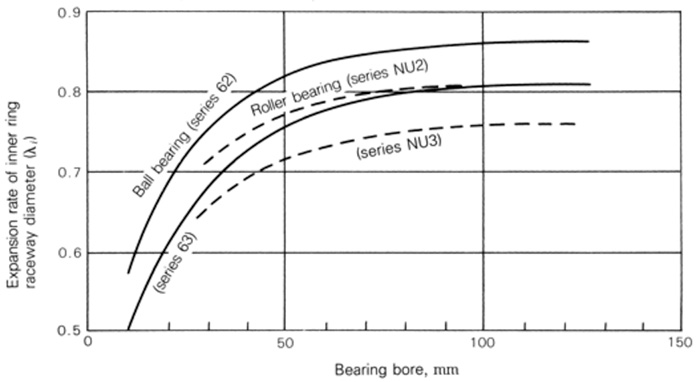Figure 2. Rate of inner ring raceway expansion (λi) from apparent interference

The average amount of raceway expansion and contraction from apparent interference is calculated from λ_i (m_m – m_i).

The following equation is used to determine, within 99.7% probability, the variation in internal clearance after mounting (R_Δf):

R_Δf = m_Δf ± 3σ_Δf = +0.014 to -0.007

In other words, the mean value of residual clearance (m_Δf) is +0.0035, and the range is from -0.007 to 0.014 for a 6310 bearing.

Radial internal clearance and temperature

When a bearing runs under a load, the temperature of the entire bearing will rise. This includes the rolling elements, but because this change is extremely difficult to measure or even estimate, the temperature of the rolling elements is generally assumed to be the same as the inner-ring temperature.

Using a 6310 bearing again as an example, the reduction in clearance caused by a temperature difference of 5^oC between the inner and outer rings can be calculated using the following equation:

The following equations are used to calculate the outer-ring raceway diameter:

Ball bearings: D_e = (4D + d) / 5
Roller bearings: D_e = (3D + d) / 4

Using the values calculated for Δ_f and δ_t, the effective internal clearance (Δ) can be determined using the following equation:

D = D_f – dt = (+0.014 to -0.007) – 0.006
= +0.008 to -0.013

Referring to Figure 3, one can see how the effective internal clearance influences bearing life, in this example, with a radial load of 3,350 N (or approximately 5% of the basic load rating). The longest bearing life occurs under conditions where the effective internal clearance is -13 μm. The lowest limit to the preferred effective internal clearance range is also -13 μm.

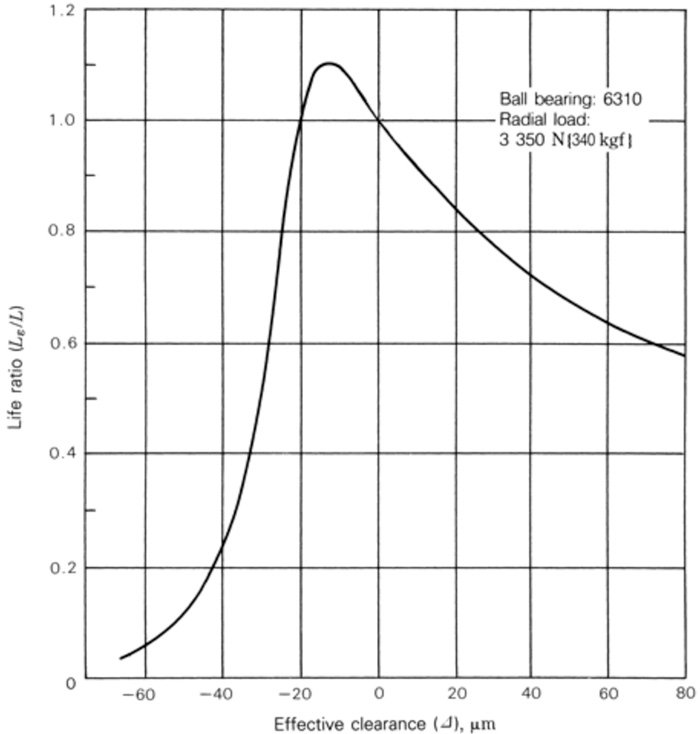Figure 3. Relation between effective clearance and bearing life for 6310 ball bearing

Application

While targeting a slightly negative clearance in theory is optimal for bearing life, in practice one must be careful when designing or building a pump with bearing preload. As shown in Figure 3, the Life Ratio does peak at -13 μm, but drops dramatically with additional preload. Incorrect assumptions regarding machining tolerances or operating temperatures can easily result in a shorter life than anticipated if the bearing becomes preloaded too heavily. On the other hand, too much clearance can result in bearing slipping and poor pump performance. One must evaluate the trade-offs of clearance and bearing preload based on the needs of the application.

Understanding the importance of bearing internal clearance will help increase bearing life and optimize your overall pump performance. For more information please contact NSK.